Forms of Movement
If you read all the pages on this website then you are likely to notice that there are several radically different ways in which a fluid, and vortices within a fluid, can exhibit movement. There are several forms of movement, essentially different from one another, that a vortex can produce. Under the influence of some of these forms of movement the vortex changes shape, for other forms of movement the overall shape of the vortex stays just about the same. The majority of these different forms of movement are forms of contraction or forms of expansion but some forms of movement do not appear to fall within either of these two categories. Some forms of movement involve other objects besides the actual vortex that creates the movement. It is important to understand the difference between all the known forms of movement and it is important to comprehend what kind of movement is referred to when a phenomenon is being described and explained.
The different forms of possible movements will be presented here on this page. All these movements apply to vortices, but some of them also apply to fluids that are in a state of equilibrium, thus volumes of fluids that do not show any vorticity (including both rotational and non-rotational vortices). A fluid for example can also change over time in its behaviour and appearance through chemical reactions or by a change in pressure or temperature. These changes can result in a change in dimensionality without the presence of vortices. The naming conventions on this page focus foremost on the movements exhibited by vortex types. For the forms of movements exhibited by fluids without vorticity the naming conventions might still be lacking. Over time I will add more forms of movement with their appropriate naming convention to this page.
Naming Conventions
All forms of movement that have so far been identified are listed in the table below. All these movements will be discussed in more detail within the next paragraphs. You should be aware that these naming conventions are mostly unique to this website and the infinity theory, these are not naming conventions used by other sources within the scientific community. I had to come up with these new naming conventions because the theory that is presented is new and it demanded these new conventions to make the theory explainable and avoid any possible mix-up and confusion. Over time these naming conventions might be altered when more people become involved with the theory and bring forth their arguments for required changes.
| Form of vortex movement | Naming convention for each of both directions | Description |
| Flowing | Pushing and Pulling | This is the movement that a vortex creates by pushing or pulling matter in the direction of the sum movement of its meridians. This movement of the vortex is the projected movement of a propagating wave. One other way to describe these two movements is by stating that a vortex exhibits a pushing flow or a pulling flow. |
| Dimensional Drifting | Drifting Upwards and Drifting Downwards | This is not the movement of the vortex but the movement of a much smaller object that is pushed or pulled within the flow of the larger vortex. It is only when the object is small enough to be contained as residential matter that this movement is called drifting downward or drifting upwards. |
|
Non-dimensional Drifting |
Drifting Forward and Drifting Backwards | This is not the movement of the vortex but the movement of a smaller object that is pushed or pulled within the flow of the larger vortex, without the object being small enough to be pushed or pulled towards the higher or lower dimensions. These are the same words we commonly use when a boat is drifting on a stream. |
| Change in Peripheral Volume | Swelling and Deswelling | A change in the volume of the peripheral shape of a vortex so that its shape appears thicker or thinner. This is a result of the vortex moving further away or moving closer to the EM spectrum region. |
| Scaling | Growing and Shrinking | Both the wavelength and the amplitude of a wave increase or decrease, thereby the wave progresses to a larger or smaller scale size. The result is that the vortex changes in size without this increase or decrease of size involving a change in shape (so far this form of movement has only been observed with bubble type vortices). |
| Morphing | Morphing Upwards and Morphing Downwards | Any form of morphing in which the shape of vortex changes from one vortex type to another vortex type because the vortex moves towards the higher or lower dimensions. Upward morphing exists, but downward morphing has not been observed and might not exist. |
| Sequential Morphing | Morphing Upwards and Morphing Downwards | A type of morphing in which a vortex changes into a subsequent vortex within the same cycle. In most cases this is also a vortex from the same class. This is due to an increase (this movement exists) or decrease (this movement perhaps does not exist) in amplitude of the wave. |
| Dilative Morphing | Dilating and Dedilating | A type of morphing that involves a movement towards to a subsequent cycle instead of a movement within the same cycle. Hereby the vortex dilates outward (this movement exists) or does the opposite movement (this movement perhaps does not exist). |
| Shifting | Shifting Upwards, Shifting Downwards | Special type of morphing in which the environment morphs because of the movement of the observer towards the higher or lower dimensions. The word shifting is thus used for the apparent movement of the environment. The word drifting is used for the movement of the object that performs the movement that makes the observed shifting possible. |
| Displacement | Moving Left, Right, Up or Down or any other direction | The whole vortex moves to a different location without this movement involving a change in shape. This is thus a common displacement movement, an object that travels from position A to position B. |
| Rotation | Rotating Clockwise or Counter-Clockwise | The whole vortex rotates to a different orientation without this movement involving a change in shape. This is thus a common rotational movement in which an object changes its orientation from angle A to angle B. |
Translations and Rotations
Contraction and expansion always correlates with a movement in the onefield. Yet what we directly observe when we are able to see any of the effects of this contraction or expansion with our eyes is a movement, an alteration, within our physical world that we do not intuitively link to a movement in the onefield. The result that we see in our perceived three-dimensional physical world is a different movement then the one that actually happens within the infinite dimensional onefield. We need to learn the connection between what we see and what it actually means in relation to the onefield and its infinite number of dimensions. All forms of movement within the onefield fall into at least two different categories, which are translations and rotations. The different angles of the axes about which these movements take place make it possible for these movements to exhibit a large variety of effects within our physical world, far more than two. Different translations give rise to different phenomena. Different rotations also give rise to even more different phenomena.
A translation in the onefield is a movement in a straight line from position A to position B within the onefield that correlates with a change of the position within the transformation sequence. A vortex that makes such a move will look and behave differently in position B compared at how it looked and behaved when it was still at position A. A translation within the onefield does not mean that the vortex makes a displacement within our visible world. In most cases the observed effect is that the vortex changes its shape, or the vortex exhibits movement without changing the actual position that we are able to see.
|
translation |
rotation |
 |
|
A rotation in the onefield is a rotation around an axis from angle A to angle B within the onefield that correlates with a change of the position within the transformation sequence. A vortex that makes such a move will look and behave differently at angle B compared at how it looked and behaved when it was still at angle A. It is not known if the axes of rotation are arbitrary axes or some sort of unit axes, it might be that some rotations take place around unit axes while other rotations take place around arbitrary axes. A rotation within the onefield does not mean that the vortex rotates within our visible world. Here too in most cases the observed effect is that the vortex changes its shape, or that the vortex exhibits movement without changing its actual orientation.
The disks (vortex class) within the second cycle of the transformation sequence exhibit a looping flow movement. Intuitively we could assume that this rotational movement should correspond with a rotational movement in the onefield because a translation can not result in a loop. However, in the onefield a rotation can not result in a loop either, this is because the number of degrees (or whatever unit we should use) of a rotation around any axis is also infinite, there is no such thing as a 360 degree looping rotation. It is therefore the most likely scenario that the projection method creates the illusion of a rotation, but this is not completely certain. It might also be that a combination of movements in the onefield can also result in a rotation, one that is real in the onefield and within our percepted world. One of the clues that hints that the movement is not really looping in the onefield is that a disk is a dilation of a spiral, which has been dilated by only changing the orientation of the projection, the path of movement should therefore still be continuing indefinitely in one direction, like when spiraling inward or outward, it now appears to loop because this endless path overlaps itself.
| Form of vortex movement | How this corresponds to movement in the onefield |
| Flowing |
Inward or outward movement of a wave which is more directional when the vortex is higher dimensional but is most directional within the onefield with its infinite number of dimensions, thus translation |
| Dimensional Drifting | Translation |
| Non-dimensional Drifting | Mostly translation + rotation, sometimes one of both |
| Change in Peripheral Volume | Translation |
| Scaling | Translation |
|
Sequential Morphing (without the usual scaling) |
Rotation |
| Dilative Morphing | Rotation |
| Shifting |
Assumed to be same as sequential morphing (but that is not certain, this might also be same as dilative morphing) |
| Displacement | Translation |
| Rotation | Rotation |
When an alteration of the shape of a vortex does change its path of movement only minimally, that alteration is most likely a result of a translation within the onefield. When the path of movement of a vortex, and thus its overall geometrical shape, changes drastically (particularly the difference between the low end and high end of a vortex), then that alteration is most likely a result of a rotation in the onefield.
It is difficult to try to assess how a displacement as a movement within our visible world corresponds to a movement within the onefield. Such a movement does not appear to correlate with a change of the location within the transformation sequence. A vortex that makes such a move can look and behave the same in position B as it does in position A. We have known for centuries how to describe and measure movement within our visible world by using the Cartesian coordinate system, yet now that we are beginning to understand the first few basics about the onefield it seems the most difficult to describe is now what was once the simplest to describe.
Because the onefield is assumed to be a medium that is comprised of an infinite number of dimensions, it is understandable to assume that the number of axes along which translations can take place and the number of axes around which rotations can take place are both infinite. However, so far only a few possible translations and also only a few possible rotations have been identified. This suggests that the range of axes that are utilized to influence observable phenomena within our physical world might be quite limited. On the other hand, the range of vortices that sequentially morph from one to another is very large, which indicates that the range of rotation around an axis should also be very large, and should correlate with angles way above 360 degrees. Besides that, there are still many more forms of movement waiting to be found and identified. The total number of translations and total number of rotations can both be far more than the number of axes within a three-dimensional Cartesian system, which is a total of three.
Flowing
The most obvious movement that a vortex can make that we at many times are able to observe is the movement called 'flowing'. It is the one movement where a vortex does not change shape, but performs a movement along the path of its shape. An analogy would be a stream of water or a waterfall. Although the stream of water does not alter much in appearance, there is a constant movement pushing the water volume forward. The shape of a waterfall stays not far from the same as the water continuously plunges downward. We see a continuous flow of movement along the length of a waterfall just as we often see a flow of movement along the length of a vortex. Flowing is sometimes a form of contraction or expansion, while at other times it is not a form of contraction nor expansion. The effects of the movement depends on the vortex type.
_Edited_UnderCC01.0.gif)
The vortex flow form of movement only applies to vortices. But the word is also commonly used to describe other flow phenomena within our daily world, such as streams and waterfalls. When using the word 'flow' as in 'vortex flow', then it is used for both rotational and non-rotational vortex types. And it is used for vortices that flow towards the higher dimensions, flow towards the lower dimensions, or vortices that only flow at a constant dimensionality value (thus no contraction or expansion).
Expansion that occurs as result of a flow movement is called pushing, and contraction as a result of a flow movement is called pulling. When a flow movement does not result in a change in dimensionality then the movement might also called pushing (such as the movement observed with the belts). In most cases it is not that difficult to determine whether a flow movement results in a contraction, expansion or none of both. For this one needs to look at how the thickness of a vortex compares at the start of its path to the end of its path. An increase in thickness correlates with an increase in dimensionality, thus an expansion. A decrease in thickness correlates with a decrease in dimensionality, thus a contraction. Another way to determine would be to look at if the vortex performs an outward movement or an inward movement. This second method can be deceiving when attempting to evaluate the movement of the revolute. The revolute appears to spiral inward but the rotation of the spiral arms starts with an outward movement, it is therefore an expansion and not a contraction.
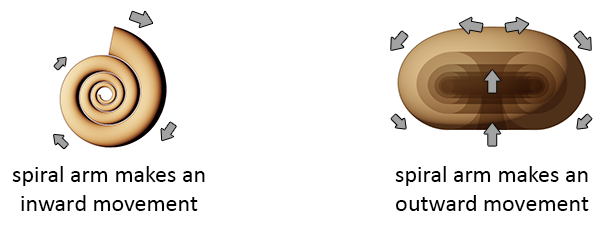
A spiral can flow, but a spiral can also rotate. Both movements are substantially different and it is important to be aware that these are two different forms of movement. When a spiral flows, the spiral arms do not move, instead the movement flows in the direction of the spiral arms. When a spiral rotates, the spiral arms do move, and the entire spiral rotates around the center of the core. The movement that hurricanes and spiral galaxies make might be a combination of these two movements but they're both at least comprised of a flow movement.

Drifting
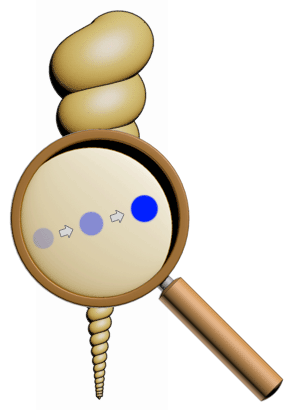
Drifting is the movement of an object or vortex caused by the flow movement of another vortex which is in most cases larger then the object or vortex being pushed or pulled. Drifting and flowing are therefore two different forms of movement that are closely related to one another. Flowing is about the object that is pushing or pulling while drifting is about the object being pushed or pulled.
Some vortices have two ends that have a different dimensionality, these are the spirals and the ladders. Movement from one such end of a vortex to the other end of the vortex results in an increase or decrease in dimensionality. This means that matter that is present within that vortex can be pushed or pulled towards the lower or higher dimensions as it moves along with the flow of the vortex. But this is only true for objects or vortices that are very, very small compared to the size of the vortex that contains them. Matter that can be influenced in dimensionality in such a way must be microcyclic in size when the vortex is mesocyclic in size, and must be mesocyclic in size when the vortex is macrocyclic in size.

Image by Varun Kulkarni
As a comparison, if you look at the swirls in the water on the surface of a pond, and see small plant particles or other small objects being pushed or pulled by those vortex currents, then you should know that those vortex currents do not influence the dimensionality of those visible objects. Although a small dust particle can be much smaller than a swirl in the water, it is still much too large to be able to travel towards the lower dimensions within the flow of that vortex. But when we compare the size of our sun, earth and other planets with the size of our milky way galaxy, then this influence in dimensionality does take effect. Our star system is constantly being pulled to the lower dimensions by the large vortex that we call our milky way galaxy, because it is millions times bigger than our star system.
Residential Matter
Whenever a small particle or object is contained in a vortex in a way that it is influenced in dimensionality by the flow of the larger vortex, then we say that the particle/object 'resides' within that vortex. Particles or objects that are influenced in such a way are called residential particles or residential objects. The form of drifting of such particles or objects or even vortices as a result of the flow movement of the much larger vortex is called dimensional drifting. An object that is being pushed by the flow of the larger vortex, thus pushed to move towards the higher dimensions, is said to be drifting upwards. An object that is being pulled by the flow of the larger vortex, thus pulled to move towards the lower dimensions, is said to be drifting downwards. This form of movement only applies to vortices that exhibit a change in dimensionality along the path of flow. It does for example not apply to looping vortices such as disks, even if the objects that reside in those vortices are small enough to labeled as residential matter.
Objects that are pushed by the flow of another vortex without moving towards the higher or lower dimensions are said to undergo a non-dimensional drifting. This happens for example when the object that is pushed by the flow is too large to undergo dimensional drifting. Such example can be a ping-pong ball being pulled by a whirlpool vortex created by unplugging a sink. Dimensional and non-dimensional drifting are two forms of movement both caused by the same other form of movement, but the difference between these to forms has huge consequences. Notice that non-dimensional drifting is also a form of displacement, which is listed at the bottom of this page.
Change in Peripheral Volume
Some vortices only consist of a core, other vortices only consist of a periphery, and some vortices have both a core and a periphery. When a vortex has a periphery, then this periphery can sometimes be more voluminous or less voluminous then what is assumed to be the most basic shape for that type of vortex. What is assumed to be the most basic shape for a particular vortex type is what lies exactly on the sine curve of the transformation cycles. When a vortex moves more away from the sine curve towards towards the midline of the curve, thus towards the region of the EM spectrum, then the volume of the vortex, which is the body of matter around the core line of the vortex, will decrease. When a vortex moves more away from the sine curve but now more away from the midline of the curve, thus further away from the region of the EM spectrum, then the volume of the vortex will increase.
It is assumable that a vortex whose shape is given its form by being located exactly on the sine curve of the transformation cycles is more stable then a vortex that is located away from the curve. It takes extra energy to extend the lifespan of a vortex that deviates from its usual, most desirable form.
The drifting form of movement only applies to vortices, and it can be any type of vortex, rotational, non-rotational, vortices with two unequal ends or looping vortices. An increase in physical volume is called swelling. A reduction in physical volume is called deswelling. When a vortex is located completely on the midline of the curve then the vortex consists only of a core, and does not consist of any periphery. Such a vortex is completely electromagnetic and does not consist of any physical matter.
When a vortex moves from the positive side of the sequence, thus above the midline, towards the lower side of the sequence, thus below the midline, its volume will flip from being positive to negative. One can interpret this type of alteration-inducing movement as a vortex that flips its body inside out. Above the midline the vortex exists in a non-plasma state, such as a gas, liquid or solid. Below the midline the vortex's peripheral body consists only of plasma.
Scaling
Scaling is when a vortex changes its size, without changing its overall shape. An increase in size is called growing. A decrease in size is called shrinking. A larger size is higher dimensional, which results in slower movement and lower density. A smaller size is lowerer dimensional, which results in faster movement and higher density.
This form of movement only applies to vortices, and theoretically it can happen with any type of vortex, rotational, non-rotational, vortices with two unequal ends or looping vortices. However, so far this form of movement has only been observed with bubbles, which are currently classified as being exterior tunnel type vortices. The notion that this form of movement has not been observed with most types of vortices might be explained by that this form of movement takes too much energy to perform. There is some type of counter-force that prohibits a common occurrence of this form of movement.
Sequential Morphing
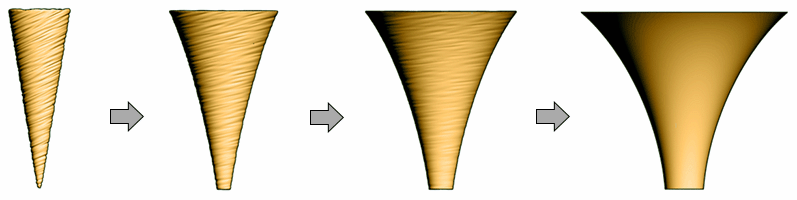
The vortices that belong to one and the same class are ordered from low dimensional (yang) to high dimensional (yin). Every next vortex is located two dimension levels higher in the sequence than the previous one. In a connected sequence the gabs between these vortices are connected by vortices of other classes. For instance, ladders connect to spirals. But besides connecting to other types and exhibiting a flow movement, vortices can also perform a movement towards the higher dimensions in a very different way. One vortex of a class can change into a subsequent vortex within the same class by gradually altering its appearance. This phenomenon is called morphing. A ladder can morph into a subsequent ladder and a spiral can morph into a subsequent spiral.
How this form of movement can be interpreted within the sine curve of the transformation cycles is that a vortex moves its position from a location on the curve to a position further upwards on the curve. The entire morph sequence for sequential morphing, which could consist of an endless number of steps, follows the exact same path of the transformation cycles.
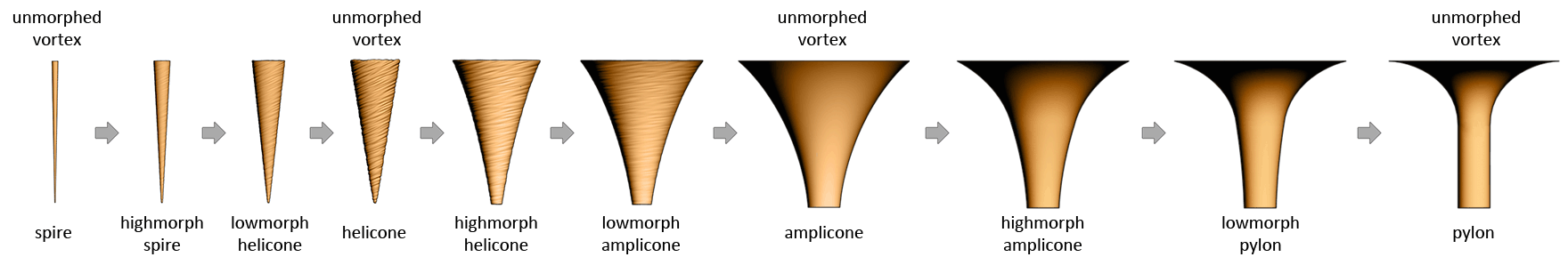
Highmorph and Lowmorph Vortices
So a vortex can be of one type but can also be an intermediate state between two vortex types, type A and type B, if it is partly, not completely morphed into another type. When a vortex is partly morphed into a subsequent vortex higher in the sequence, and still looks in appearance most as the lower dimensional vortex, type A, then we say that the vortex is a highmorph vortex of type A. When the vortex is morphed a bit further into the sequence, and looks in appearance more as the higher dimensional vortex, type B, but not completely because its appearance is still influenced by the shape of the first vortex, then we say that the vortex is a lowmorph vortex of type B.
When a vortex is not a highmorph or lowmorph but is a vortex of one type only in its plain form, then we say that this vortex is an 'unmorphed' vortex. So you can have an unmorphed spire or an unmorphed disc etc. This process called morphing will be explained in more detail on the page about morphing.
Upmorphed and Downmorphed Vortices
A slightly different term from highmorph and lowmorph is upmorphed and downmorphed vortices. Which means the same except for one significant difference. When a vortex is a highmorph or lowmorph vortex this does not mean that the vortex has actually changed shape. And there's also no indication of a direction of such movement. It is possible that a vortex exists in an intermediate state of two vortex types throughout its life cycle without altering its appearance further towards the higher or towards the lower side vortex. In that case we should only use the terms highmorph and lowmorph. We should also use these terms when we are uncertain if the vortex is or has altered.
But when a vortex has actively morphed from a lower dimensional vortex into a higher dimensional vortex then we can say that the vortex has upmorphed. And we say that the vortex is an upmorphed vortex. If the movement goes in the other direction that we can say that the vortex has downmorphed. And such a vortex is called a downmorphed vortex. Note however that morphing in the direction of the lower dimensions has not yet been observed. So far all the morphing appears to progress in the direction of the higher dimensions (along with a gradual increase in size). But just in case we do observe this later on the terminology for that is already available.
These naming conventions only apply to vortices, and it can apply to both rotational and non-rotational vortices. But this direction of movement itself can also occur with fluids that do not exhibit vorticity, yet here this form of movement is not called morphing because these are no shapes visible that change into other shapes. There is a whole page dedicated to this form of movement, the page about morphing, which can be found further ahead.
Dilative Morphing
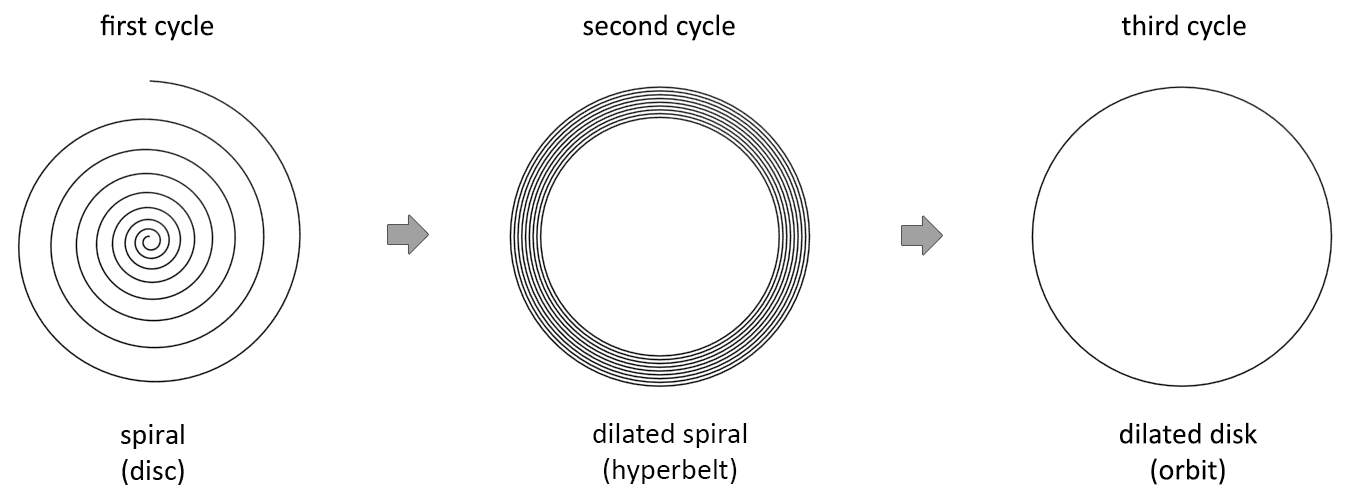
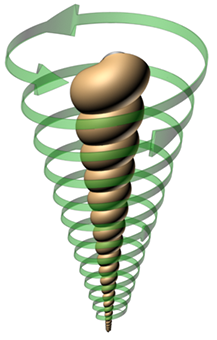
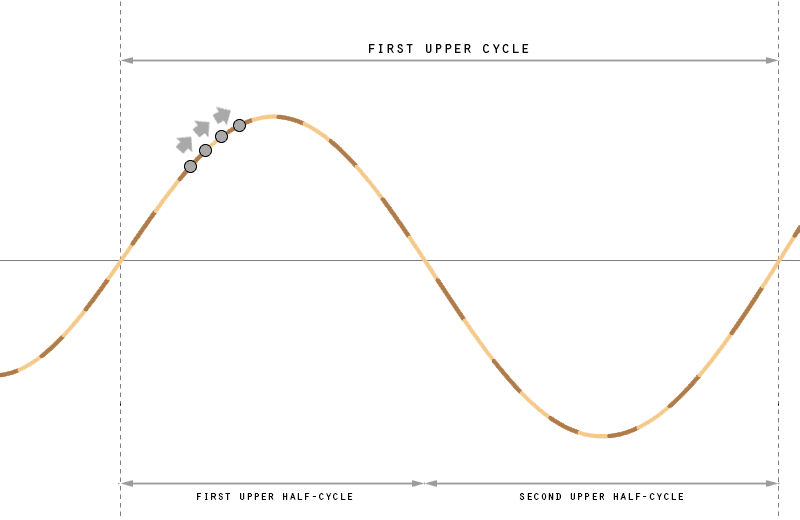
While sequential morphing is the gradual transformation of a vortex into the next vortex of the same class within the same cycle, dilative morphing is the gradual transformation of a vortex to the vortex positioned at closest proximity to the first vortex within the next cycle of the sequence. An example of such a direction this movement can take place within the transformation sequence is shown within the animation below. The image shows the different cycles of the transformation sequence laid out in a spiral, one rotation of the spiral is one cycle in the sequence. Movement along the direction of the spiral is called sequential morphing, the vortex morphs into the next vortex in the sequence of the same class (two dimension levels higher for each step). Movement from one cycle to the next is called dilative morphing, which is a distinctly different type of morphing.

The visual effect, the result so to speak, of the dilative morphing of a vortex is that the peripheral body of the vortex together with its core line is pushed outwards from the center point or center axis of the vortex (this axis can also be bend). The vortex becomes more empty at the center and more condensed at its outwards regions.
Just as with sequential morphing, dilative morphing only applies to vortices, and it can apply to both rotational and non-rotational vortices, yet it is not fully understood yet how non-rotational vortices dilate. In the previous movements discussed on this page the helicone is used as an example, which is the cone shaped vortex that looks like a seashell. Here the disc spiral is used instead because it is not known yet exactly how a helicone dilates.
The same direction of movement can also occur with fluids that do not exhibit vorticity, such as a fluid that transitions towards a vacuum, yet here this form of movement is not called morphing because these are no shapes visible that change into other shapes.
Shifting
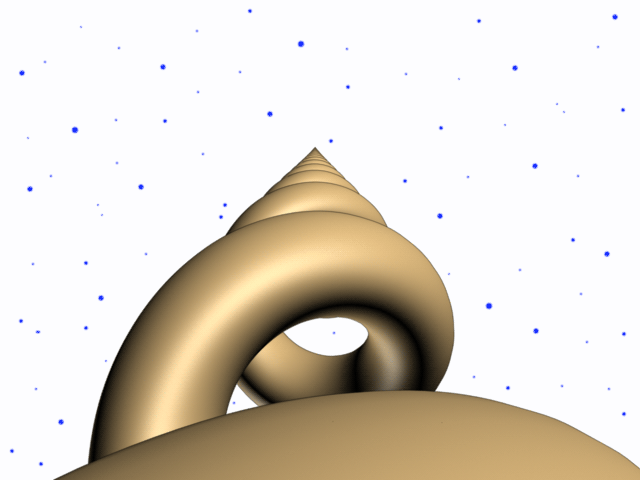
This form of movement is an effect of another form of movement, which is dimensional drifting. When an object that is mesocyclic in size resides within a vortex that is macrocyclic in size, then the flow of the macrocyclic vortex can push or pull the smaller object towards the higher or lower dimensions. This can also happen when the larger vortex is mesocyclic in size, and the smaller object or particle is microcyclic in size. So if we ourselves reside within a large macrocyclic cone-shaped vortex that exhibits a flow movement from the lower dimensions towards the higher dimensions (from the narrow end of the vortex towards the wider end of the vortex), then we ourselves would be traveling towards the higher dimensions.
But as we travel towards the higher dimensions, we do not experience a dimensional change in our own environment or our own physical self. We ourselves or the objects closely around us do not start to look or behave differently. This is because dimensionality is always relative, and our perception of it is determined by the difference in dimensionality of our own environment with that what is in front or behind us within the flow of the much larger vortex in which we reside. What we do experience is that the whole universe around us changes in appearance because of our own movement within that helix-rotational cone vortex. We would see the galaxies in the sky appear to be moving towards us (but they would do so in a relatively slower and slower rate). The galaxies would also change shape, they would morph into other vortex types. But this change in appearance of the sky is a very slow gradual change that takes many, many years.
The change of the environment that an observer who is currently drifting downwards or upwards can see through his eyes or through the lens of a telescope is called shifting. As a result of our own movement the universe around us can shift upwards or downwards, thus expand towards the higher dimensions or contract towards the lower dimensions. Shifting is what can make the universe around us change as a direct result of ourselves traveling into a specific direction of the onefield (this direction can also include a gradual change in angle). This form of movement applies to both objects residing within vortices or objects residing within a fluid without vorticity that changes through chemical reactions or other factors. More information about this form of movement will be presented on the page about morphing, and the page about time.
Displacement
One of the last forms of movement to mention is simply called moving or displacing. This is the only form of movement that is not a form of contraction or expansion. By saying that a vortex moves or displaces itself I simply mean that the position of the vortex changes within the environment, without the shape of the vortex changing radically (unless it happens simultaneously with another form of vortex movement). This is for example the movement of a tornado from one side of the road to another, or the movement of a hurricane from over water to over land. There can be all kinds of deformations along the way but these are not per see caused by the displacement itself, these alterations also can happen when such large vortices are standing still.
At first it might seem pointless to mention this form of movement on this page. There is however a good reason that makes it important to mention it and that reason is for not getting things mixed up because of the lack of terminology. I need to be clear about the words that I use so to avoid confusing anyone about what I mean when I talk about a specific form of movement. So it is worthwhile to mention this most common form of movement that is not really a movement that is specific to vortices but can happen to any object.
Rotation
This last form of movement falls in the same category as a displacement movement as by the fact that it involves only a common movement of an object that can be described by a simple movement within a Cartesian coordinate system. This is simply a rotational movement of a vortex so that the vortex changes its orientation, without the vortex itself changing its shape. One example of this was already mentioned further upwards on this page, on the section about flowing, shown by the rotating spiral. With vortices that also have a rotational flow movement it is not always easy to distinguish between the flow movement and the rotational movement, that is something one needs to be aware of.
Now that some of the basic forms of movement that vortex types can exhibit have been described, let us now look at the actual vortex types. The next pages describe a large number of different vortex types, categorized into a smaller number of different vortex classes. Each page will describe one new vortex class.
| All content on this site that is authored by Peter.A.Venis is licensed under the Creative Commons CC BY 4.0 license, unless otherwise mentioned. Most pages on this website also include material from other authors, under different licenses. Both the author's names and the licenses are mentioned in the file names whenever this information is known and can be provided. The presence of work from other authors on this website does not necessarily imply that those authors endorse the contents of this website. |
| A word that is often used on this website is the word 'vortex'. Many sources describe a vortex as a movement in a fluid that has a rotational flow. Yet many of the vortices that I describe on this website do not show a visible rotational flow. I took the liberty of using the word vortex for describing a phenomenon that had not been understood before, one that links together rotational and non-rotational movements. Even a movement in a straight line can in some cases be categorized as a vortex, if it is known that that movement is created by certain identical conditions. So keep in mind that the word 'vortex', within the context of the infinity-theory, has not the exact same meaning as other sources describe. |